Interest seems like a boring topic. Perhaps even a hard topic. Actually, interest is easy. And once you see how interest works, you will be both surprised and well equipped to make simple, smart decisions.

If you take out a 30 year mortgage for a $200,000 house at 6% interest you will pay a total of $431,676.38. A staggering $231,676.38 of that in interest alone. You’ll pay more in interest than you will for the house itself. And 6% is historically a very good rate.

The average American moves every 7 years. If you had the house for only 7 years and sold it, you would have paid over $80,000 in interest and you would still owe $179,278.77. In other words, after 7 years and $80K in interest payments, you would have paid off less than $21,000 of the $200,000 loan, or just barely over 10%. Seven years is almost a quarter of the way through the 30 year loan, yet you would still owe nearly 90% of the loan to the bank.

Think about that: average house, $80K in costs to borrow $200K for 7 years. And that is at a reasonable 6% annual interest rate.

How are these numbers derived? Are lenders just ripping us off? Is there anything you can do?

It turns out—surprisingly—lenders are not ripping you off, at least not at this nominal, text-book level. Its a free country, and, within reason (and banking regulations and predatory lending laws), anyone can lend you money at any rate and you are free to accept or refuse.

Indeed, there is competition driving rates down, and so if 6% is such a good rate, then why does this all seem like such a raw deal? And if 6% is good, what about the 18% your credit card is charging?

When banks advertise an interest rate, they advertise an annual rate^*. A percent—from the Latin per centum—is expressed as part of a hundred, so 6% means 6/100 = 0.06. For every $1 you borrow, you’ll pay 6 cents to the bank each year you owe them that $1. That does not sound so bad; but things add up.

A loan of $200,000 at 6% with annual payments means that after one year, you will pay 0.06 x $200,000 = $12,000 as the "interest" or cost for borrowing the money.

In practice, banks will not allow you make one annual payment every year, they require a payment each month. So instead of 6% year, they use (6% / 12 months) each month, or 0.5% per month. That's one half of one percent, or (0.5 x 1/100) x $200,000 = $1,000 per month.

So every month that you owe $200,000, the bank will charge you $1,000.

Congratulations. You now know almost all there is to know about interest rates. It really is that simple. And while there are a myriad of "mortgage products" that add complications, at their core they are all based on this simple, reasonable, foundation. Your mortgage statement may not look this simple, but we'll explain why and see that it really is based on this simple application of monthly interest.

So how do we get the crazy numbers of $80K over 7 years above?

If you had an interest-only loan—a loan where you only paid interest—then we are done. You will pay the bank $1,000 every month, every year, for eternity. You’ve just become the bank’s best friend because you never pay off the loan, you always pay them: better than a slot machine in Vegas, because a slot machine sometimes pays and (mostly) doesn’t. But you will pay—a $1,000 month—for eternity.

Credit card issuers also know this. They love to give you a low minimum monthly payment, because the less you pay off your loan, the longer you will pay them, even if you never use their services again. That’s not a good deal for you. Remember: a minimum monthly payment, or an "affordable" monthly payment that the auto sales person so "kindly" arranged for you, may be a convenient service, but it is rarely the best deal for you. At the extreme, the lowest monthly payment is an interest-only payment, that is the worst deal for you because you will never pay off the loan. (Actually, believe it or not, there are arrangements that are even more expensive: some loans can be arranged with so-called negative amortization. Each month you pay less than even the interest due, so the balance due builds each month [you pay interest on interest]. Clearly, these are, sooner-or-later, unsustainable).

In these situations you are an indentured servant.

No one can pay forever, so interest-only loans are a special case. Often the interest-only period is for a specified time and then it converts into a loan that forces you to pay off some of the underlying principal—eventually all of it.

---

^*Lenders often advertise an APR (Annual Percentage Rate), which is slightly higher than the loan's nominal annual interest rate. The APR includes the effects of some of the loan's other costs, such as some closing costs, into a new, effective rate. You are not charged this effective rate per se; it is calculated to help you compare rates where different loan products have different closing costs. Comparing APRs is not perfect, but for a given lender, if their APR is noticeably higher than the loan's nominal rate, then you know they are bundling in higher closing costs.

Each month, you will pay the bank exactly the interest as we have calculated it. You will take the monthly interest rate (the quoted annual interest rate, divided by 100 to turn it into a proportion, and then divided again by 12 to convert into a monthly rate), multiply it by the principal, (the amount you still owe, also called a balance) and that’s what you owe in interest that month. With the exception of various fine-print fees or "adjustments" on special cases, the interest you owe is really that simple.

Now comes the magic of amortization. Each month you must additionally pay some of the principal to help pay down the loan. But how much? For a house or a car loan, you agree up front to some specified time period; such as 30 years for a house or 3 years for a car.

Thus, there is some fixed amount (your monthly payment) that equals the interest you owe plus the payment against the principal, such that if you paid that total amount each and every month, the monthly payment would not change and you would pay off the loan in exactly the number of months agreed.

So we have a few constraints: the monthly payment does not change (that makes it easy for you to budget your expenses), the loan will be paid off in the allotted time, such as 30 years (so you are not getting into anything open ended), and each month you will pay exactly the agreed upon interest on the money owed at that time.

For every combination of principal, interest rate, and term, there is exactly one and only one number that satisfies these constraints: that number is your monthly payment.

This certainly appears like a fair way to do things. And it is. Lenders compete by lowering or raising the interest rate: the way the interest is charged and why you end up paying $80K to borrow $200,000K (40%) for 7 years on a 6% loan is not a result of banking collusion on the formula, it is a result of just what “6%” on $200,000 for an anticipated period of 30 years really means.

It turns out that calculating the monthly payment is easy. Many spreadsheets and calculators do it for you. The formula is many hundreds of years old and is well known. The formula is:

Payment is the monthly payment; Balance is the remaining balance on the loan (the amount you owe now, not yet crediting this month's payment); Interest is the monthly interest rate (so Interest = annual interest rate / 12; for example 6% is 0.06 / 12 = 0.005); and n is the total number of months for the loan (30 years is 30 x 12 = 360). (1 + Interest)^-n means 1 / [ (1 + Interest) times itself n times ].

For our example the monthly payment is:

Recall that for an interest-only loan the cost was $1,000/month. With our 6% loan at the end of the first month we have paid nothing on the loan yet, so now we will pay the bank $1,000 in interest and $199.10 against the principal for a total of $1,199.10.

The bank will receive this payment, take a $1,000 as their "interest" in exchange for lending you $200,000 for one month, and credit your balance $199.10 as the amount you also paid to pay down the principal.

Now in the second month we should pay a little less interest because we no longer owe $200,000. We paid $199.10 against the principal, so now we owe $200,000.00 - $199.10 = $199,800.90.

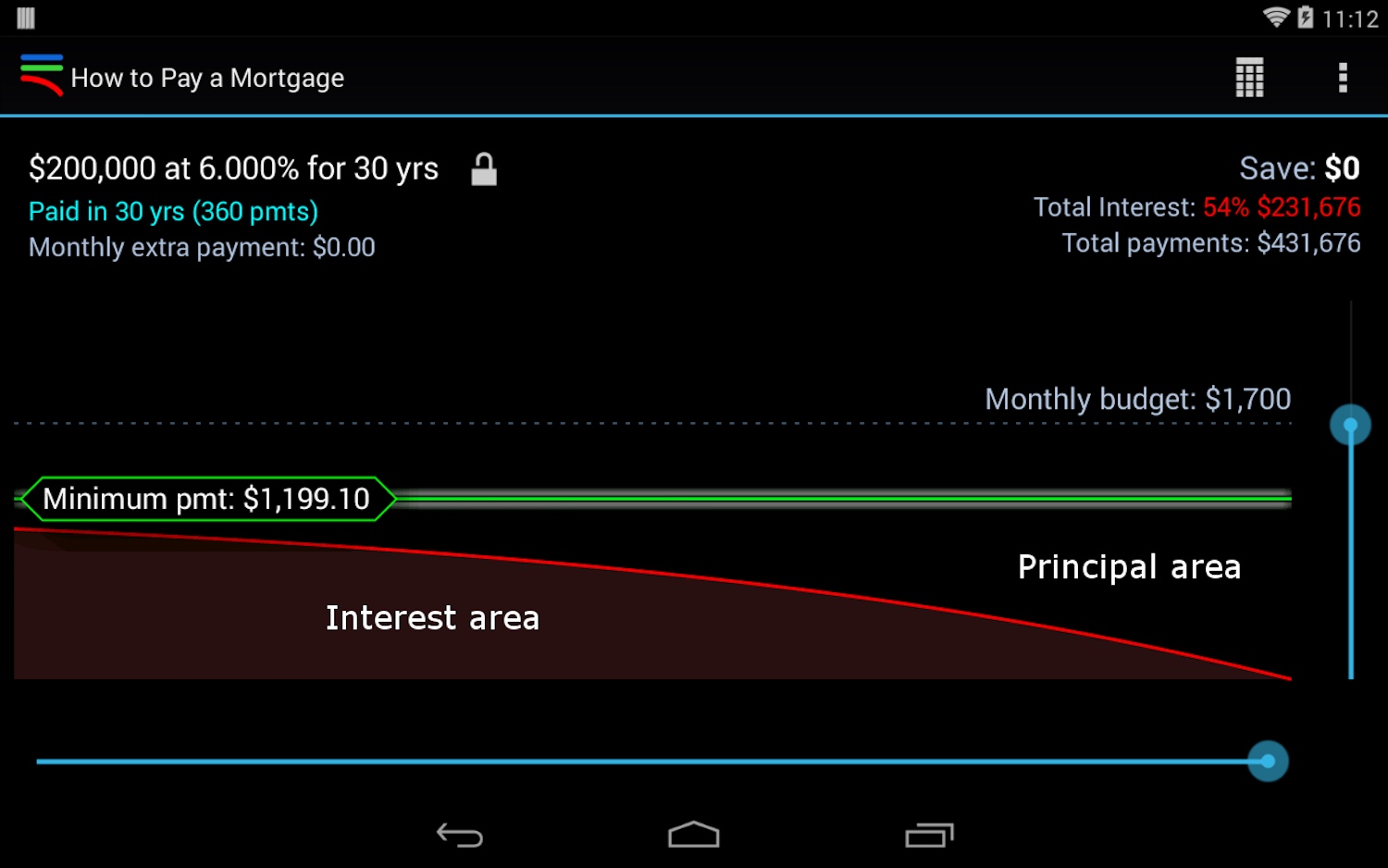
Our monthly payment amount ($1,199.10) never changes, so if we pay less in interest, more of that payment goes to principal. Here’s a graph of showing how fixed monthly payments are split between principal and interest:

From the app How to Pay a Mortgage. Red line is the interest curve. Monthly amounts are available on a separate amortization screen.

We see that in the first few years the principal is paid off slowly because most of the payment goes to interest. Indeed, for the first month we paid $1,199.10 but only $199.10 went to pay down the loan (viewable in the amortization table; not shown). By the second month the amount paid to principal is $200.10—just $1 more than the previous month. The amount paid to principal goes up a little each month, because each month the interest due is calculated on a slightly smaller balance.

What if we just took the total interest due $231,676 and divided it by the total number months 360? We would get the average interest to be paid each month ($644) in a way that we might think is “fair:” just split the total interest due and pay that each month. But we would be wrong.

By the end of the first month we would be pay $644 interest on $200,000. That’s 0.0032 of 200,000, not the agreed upon 6% annual = 0.06 / 12 monthly = 0.005. We are underpaying interest. Of course, the market won’t let us get away with this. Lenders will respond, independently, by raising the annual interest rate until the realized return on lending actually produces what the market will bear for interest on originating loans.

Now let us look at month 360—the last month of the 30 year loan. An interest payment of $644 from a monthly payment of $1,199 implies a principal part of $555. And since this is the last month, this is the entire remaining balance. So we would be paying $644 "interest" on an outstanding balance of $555: that's more than 100% for just one month! We are paying too much interest. So this incorrect model artificially creates pressure to refinance in the later years, because if we re-amortize at the same 6% with a new, shorter loan with another lender near the end of the term we’ll pay less.

Let’s refocus on the correct amortization model to understand why loans are amortized the way they are. Inspection will show that the model retains it’s properties perfectly throughout the entire length of the loan, creating no artificial pressures. For example, if we pay for 1 month, or 360 months, or any month in between, and then calculate a new loan all over again on the remaining principal with the remaining time as the new term, we’ll get exactly the same pay off schedule with exactly the same monthly payment. So there is no inherent pressure for either lender or borrower to change the terms of the loan as the loan is being paid off, all else being equal.

Amortizing correctly, in the first 7 years we pay $20,721.23 against the principal. In those same 7 years, we paid $80,003.26 in interest (an average of $952.42 per month—47% higher than our $644).

Depressing.

The shocker is that 6% interest, which doesn't sound like much, means that over 7 years we will pay 40% of the initial principal ($80,000 / $200.000 = 0.40 or 40%) in interest, and we still owe almost 90% of the loan. Yet there is no magic; it is all available for any one to calculate.

And it is fair—that is, we agreed to pay 6% annually (1/2 of 1% each month) on whatever is owed, and that's what we are doing. Indeed, if we had taken an interest-only loan for those 7 years, we would have paid 0.06 x $200K = $12,000 for each of 7 years, for a total of $84,000.

Now that is a shocker. This really tells us that the difference between 30 years and eternity (the "term" for an interest-only loan) is not that large at the beginning.

The closer your monthly payment is to the interest-only loan, the closer you are to indentured servitude. A monthly payment of $1,199.10 on a 30 year note is quite close to the interest-only amount of $1,000. So a 30 year note—about half of your expected adult life—is an expensive way to own a home.

Thirty-year fixed term mortgages offer many advantages. Not every country offers them (in fact, the U.S. is somewhat unusual in this). They absolutely can help people enter home ownership. And, all else being equal, that's a good thing.

But don't let the fact that "everyone" gets a 30 year loan, and that a 30 year loan allows you to qualify for the biggest house, suggest to you that minimum payments on a 30 year loan is unequivocally good debt for you. A 30 year loan with minimum payments is the closest you may be to indentured servitude while still maintaining a viable business model for the pay back of the principal. A 30 year loan is very expensive—and as we have seen, paying it back early (for example after 7 years) doesn't make it less expensive—in a relative sense, its exorbitantly expensive: you'll pay 40% ($80,000) just to borrow that $200,000 for 7 years.

Archimedes of Syracuse (287 B.C. - 212 B.C.) was a Greek mathematician who famously said "Give me the place to stand, and I shall move the earth." Archimedes wrote a treatise on levers, where he recognized that with a lever you can trade length or distance for force: a lever lets you move a lot of weight a small distance at one end by applying a smaller force over a large distance at the other end. Bicycle gears work on this principle, where a "low" gear allows you to move any given point on the pedal gear a longer distance with lesser force, in exchange for rotating the rear sprocket gear a smaller distance with greater force. Stairs are analogous to a "static lever" (an inclined plane), where you again trade distance (the flight of stairs) and many little steps in place of one high, immediate jump.

Levers let you use increased distance with lower effort per unit time to achieve a desired result that would be otherwise unachievable without the application of excessive force. Over the total amount of energy exerted, levers "cost" something—there is some frictional and entropic loss; but they are worth it: without them you may not be able to even get the task done.

Interest and mortgages are financial levers. But they are levers that work over time, not distance. You trade payments over time in exchange for access to a large sum of money now. And since it is presumably the fruits of your labor over time that is paying back the loan, you can think of your mortgage as converting your future work effort into a financial instrument for your benefit, now. Indeed, in the U.S. fractional reserve system, when banks lend you money, they rarely lend you "their" money. They are lending you money they access from the Federal Reserve, money which it ultimately created "out of thin air." Is that justified? Yes it is. The U.S. Federal Reserve does not "push" money; the act of borrowing "pulls" money. Under the mechanism of loans to consumers via banks, the act of money creation via loans essentially amortizes the future work value of citizens for effecting wealth creation (investment capital) today: it is using time as a lever. How better to modulate the monetary expansion of an economic society than to let the act of borrowing by the society's very participants themselves amortize their own work effort for use in investment. So when you borrow from the bank, you are essentially borrowing your own future work value monetized for use today. So of course it is "out of thin air," because your future work has not yet been done. If people borrow against their own work effort and do not invest it, but "squander" it on non-productive consumption and ephemeral activities, then caveat emptor; the system is doomed. Don't ask a fish to discover water: we cannot save ourselves from ourselves. But if we borrow wisely (Good Debt), then leveraging your own future work for your benefit today is a critical lever for a raised standard of living.

You want to use levers where you need them. But you want to minimize the friction and excess cost in their use. So while interest works as a powerful tool for you as a borrower, you still need to buy things at a good price. And that means buying money at a good price: securing the right financial lever. For a mortgage, the "cost of money," is not just the interest rate; it is the interest rate and the length—or term—of the loan. This is important and we will spend a lot of time on this point.

---

Next: One